Finally,…, in place of our Programming Clinic, we conclude this first set of lectures with a quick, largely computational approach to the Field theorems of Continua. This, combined with our earlier treatment of differential calculus, completes the application of the results of calculus to tensor objects.
[gview file=”http://oafak.com/wp-content/uploads/2019/10/Week-Twelve-2.pdf”]
Time did not permit us to look too deeply into some of these matters. Take this as a way to whet your appetite to a fruitful understanding of Continuum Mechanics. We can avoid further theoretical work and the covariant formulation by using Mathematica for our computations. We shall more of such in the next chapter where we shall begin to look at the geometric formulations of continua.
Good day Sir,
As regards the self test. Will the test affect our curative score as you said earlier, whereby the average will.be taken every Time we attempt the test?
NWANKITI UGOCHUKWU
Mechanical engineering
160404017
In the table in page 11 of chapter 3, what is the difference between the “contraction product” operation and the other operations there
When you take a trace of a tensor, you are contracting it and reducing its order by two.
Yes. If you don’t want the tests to affect your grades, don’t take them. We will ensure your grades are not reduced by taking the test. If you take a test more than once, your average scores will be used.
Good day sir,
I don’t understand how this solution from chapter 2, question 2.12 works. In particular from line 2 downwards
()^ = (det())()−T
= (^3 det())−1^(−)
= (^2 det())^−T
= ^2^c
Hello Temitayo,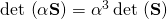
. Using this, beginning with the definition of the cofactor of 
on the first line, the rest of the proof is straightforward:
There is an error in this question. It has been corrected and may have been posted by the time you read this. In order to follow the steps here, you will need to understand from 2.42 that
(1)
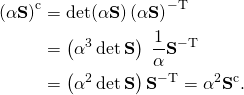
Good day sir,
Can I call the curl of a vector, the surface base vector since it is the derivative of the position vector on the surface.
Could you please indicate where you got that erroneous definition of the curl of a vector from? For the third-order Levi-Civita Tensor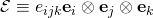
S11.36 defines the curl of a vector as 
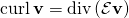. It is also easily shown that 
Okay sir
Tensor Analysis III slide 18
Alas, you misunderstand the Mathematica code (in S12.18) you are referring to. There is no point in the code where the curl of a vector is defined. Instead, the code calls the in-built definition of the curl of a vector implemented by Mathematica. That definition conforms to the definition given in the slides and repeated here.
It will be necessary for you to study and understand when a code defines and when it is a call to a defining function, as is the case here! These issues were taught in Programming Slides 1 PS1.7-11
Good day sir,
Can I call the curl of a vector; surface base vectors since it is the derivative of the position vector on the integral surface.
No. That is NOT the definition of the curl of a vector. The physical meaning has to do with the tendency to rotate. There are textbooks where it is called the “rot” for rotation. Don’t confuse the meaning of curl with Stokes theorem that provides a relationship between surface integrals of curls of vectors and line integrals of the vector.
Good day sir, can we solve the disk with the stoke theorem by making the disk a surface?
The disk is a surface, a surface only and nothing but a surface. How else did you think you can analyse the disk?
Good day Sir, I would like to know on what ground, the product between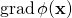
and the vector differential, 
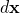, is a scalar product and how it can be proven.
1. We know that it is a product by definition. The Fréchet derivative is said to exist if and only if such a product exists between the grad and the differential of the argument that results in the Gateaux differential. The argument differential is certainly a vector because we are talking of a function defined in a Euclidean Point Space; a vector joins any two points. We also know that the differential of the function: the difference between the value at two different points, is necessarily a scalar as the difference between two scalars is a scalar (remember , we showed, S10.25, that the Gateaux Differential degenerates to an ordinary differential once the argument and return value of the function are both scalars). So you have,
(1)
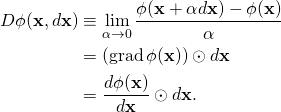
where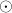
is a yet to be defined product.
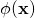, alias 
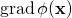or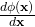 
is a vector, the only possible way its product with another vector can give us a scalar is the scalar product. The other possibilities are vector product and tensor product; neither will result in a scalar.
is a vector. One easy way is to remember that varying a scalar with respect to a vector will give us three unique scalars: each corresponding to a variation with respect to each component of the vector. Another way is to ask: What can you multiply with a vector to give you a scalar? A scalar? a second-order tensor? a third-order tensor? This absence of an alternative is sufficient reason to tell us that it HAS TO be a vector!
2. Once we accept the fact that, the Fréchet derivative of
3. There are several ways to see that